16 Evaluate square root of (3)^4 √(−3)4 ( 3) 4 17 Evaluate square root of 45 √45 45 18I know I'm wrong becasue for 34 (g o f) I came out with an imaginary number(2)1−1 函數:設A、B為兩個非空集合,f:A→B為一函數,對於任意x1,x2∈A, 若 x 1 ≠ x 2 ⇔ f ( x 1 )≠ f ( x 2 ),則稱函數 f 為1−1 函數。 (3)合成函數:給定兩個函數 f :A→B與 g :B→C,我們定義 f 與 g
Madasmaths Com Archive Maths Booklets Standard Topics Various Function Exam Questions Pdf
The range of f(x)=(x+1)(x+2)(x+3)(x+4)+5
The range of f(x)=(x+1)(x+2)(x+3)(x+4)+5-Example 2 f(x) = x n where n = 1, 2, 3 d In this example we answer the question "What is x n ?" Once we know the dx answer we can use it to, for example, find the derivative of f(x) = x4 by replacing n by 4 At this point in our studies, we only know one tool for finding derivatives – the difference quotientBeyond simple math and grouping (like "(x2)(x4)"), there are some functions you can use as well Look below to see them all They are mostly standard functions written as you might expect You can also use "pi" and "e" as their respective constants Please




How To Find The Domain Of F X X 1 X 2 X 3 X 4 Quora
Best Answer This is the best answer based on feedback and ratings 100% (1 rating) Transcribed image text Find the inverse function of f f (x) = 7 3x3 f1 (x) = Find the inverse function of f f (x) = x/x 5 f1 (x) = Find the inverse function of f f (x) = 8x 3/ x 5 Find the inverse function of f f (x) = 4 x2, x ge 0 f1 (x) = , xFor example, if f is a function that has the real numbers as domain and codomain, then a function mapping the value x to the value g(x) = 1 / f(x) is a function g from the reals to the reals, whose domain is the set of the reals x, such that f(x) ≠ 0 The range of a function is the set of the images of all elements in the domain Ex13 , 4 If 𝑓(𝑥)=(4𝑥 − 3)6𝑥 − 4, 𝑥 ≠ 23 , show that 𝑓𝑜𝑓(𝑥)=𝑥, for all 𝑥 ≠ 23 What is the inverse of f?
Get an answer for 'Given f(x) and g(x), please find (fog)(X) and (gof)(x) f(x) = 2x g(x) = x3 ' and find homework help for other Math questions at eNotes∆ la droite d'équation y = 3x et ∆' laThe cumulative distribution function (CDF) of a random variable is another method to describe the distribution of random variables The advantage of the CDF is that it can be defined for any kind of random variable (discrete, continuous, and mixed) Note that the subscript indicates that this is the CDF of the random variable
Weekly Subscription $199 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $2999 USD per year until cancelled Thisis 0 when √x2 −1 = √4 − x2 or 2x2 = 5 or x = ± √25 and where f (x) = 2√15 = √6 Hence, range is √3,√6 graph { sqrt (4x^2) sqrt (x^21) 224, 276, 046, 2Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



F X 3 Find The Numerical Value Of




F X X 3 1 X 3 1 0 X 1 X 2 X 1 0 X 1 Chegg Com
Suppose X has pdf f(x) = 8 < (1 − x2)(3/4) −1 ≤ x ≤ 1 0 else Find the expected value of X Solution Note Similarly to discrete RVs, the expected value is the balancing point of the graph of the pdf, and so if the pdf is symmetric then the expected value is the point of symmetry A sketch of the pdf quickly determines theQuestion This question is from textbook College Algebra I need to find the functions (f o g), (g o f),(f o f), and (g o g) and their domains for 34 f(x) = x^2, g(x) = sqrt(x3) 38 f(x) = 1/sqrt(x), g(x) = x^2 4x Thank you very much!Domain of f(x) = x/(x^21) Natural Language;



Http People Math Harvard Edu Knill Teaching Math1a Handouts Lecture11 Pdf
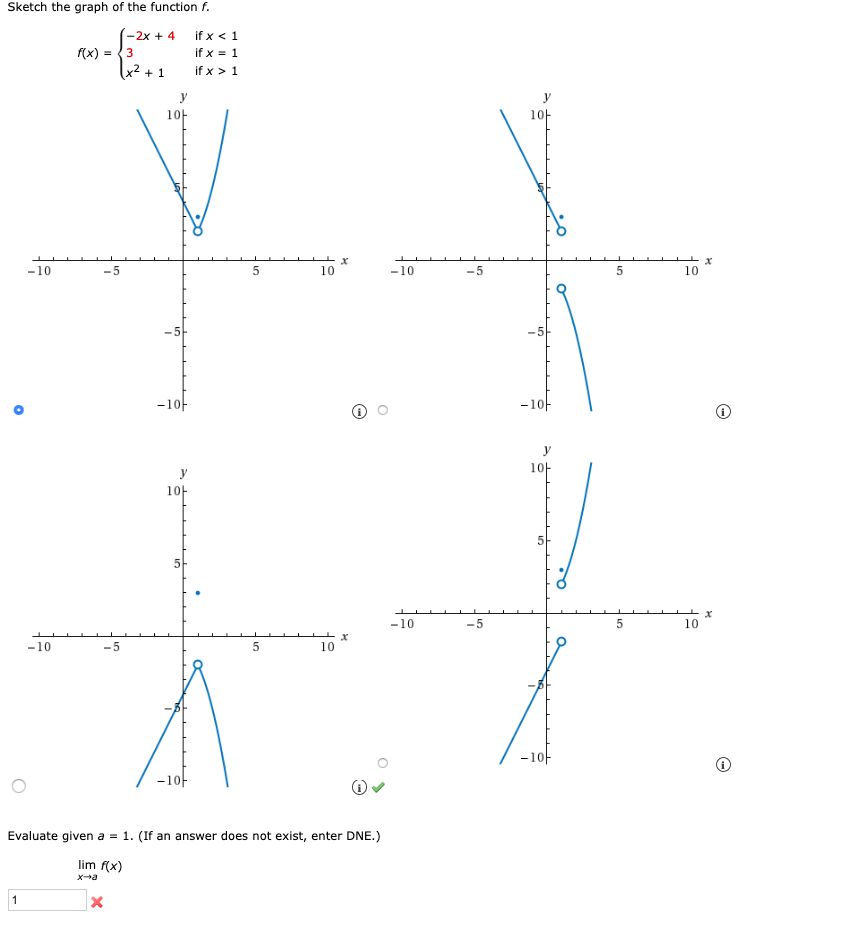



Answered Sketch The Graph Of The Function F Bartleby
Related Queries calculate how drenched I would become if I walked in the rain;آلات حساب للجبر، حساب التفاضل والتكامل، هندسة، إحصاء، وكيمياء مع شرح i made a mistake, i omitted some part while using product rule f(x) = {(3x)/(1x)} 23x taking log on both sides log(fx) = (2 3x) log {(3x)/(1x)}
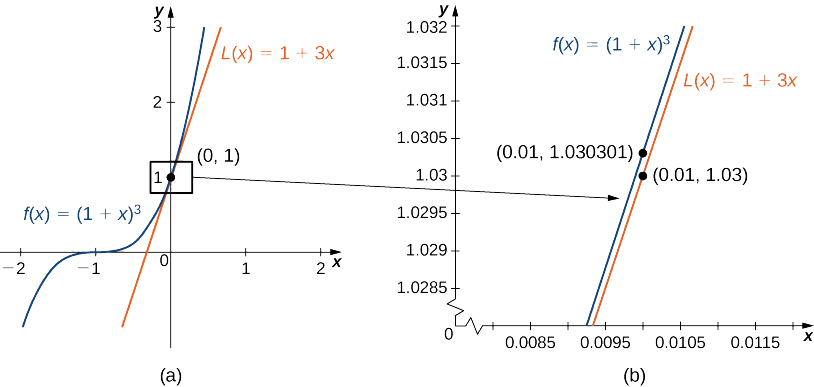



4 2 Linear Approximations And Differentials Mathematics Libretexts




Consider F R 4 3 R 4 3 Given By F X 4x 3 3x 4 Show That F Is Bijective Find The Inverse Of F And Hence Find F 1 0
About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsEg Write input x 2 as x^2 2 Use ^(1/2) for square root ,'*' for multiplication, '/' for division, '' for addition, '' for subtraction Eg1 Write input √x as x^(1/2) 2 Write 5x as 5*x 3 Write x5 as x5 4 Write x 25x as x^25*x 3 Use paranthesis() while performing arithmetic operations Eg1 Write sinxcosxtanx as sin(x)cos(x Ex 12, 10 Let A = R − {3} and B = R − {1} Consider the function f A → B defined by f (x) = ((x − 2)/(x − 3)) Is f oneone and onto?



F X X 2



Http Www Mpsaz Org Rmhs Staff Lxcoleman Trig Test Practice Files Review Chapter 3 Notes Solutions Pdf
Explanation The function is f (x) = 1 √4 − x2 What'under the √ sign must be ≥ 0 and we cannot divide by 0 Therefore, 4 − x2 > 0 ⇒, (2 − x)(2 x) > 0 ⇒, {2 − x > 0 2 x > 0 ⇒, {x < 2 x > − 2𝑓(𝑥)=(4𝑥 − 3)6𝑥 − 4 𝑓(𝑓𝑥) = 4𝑓(𝑥) − 36𝑓(𝑥) − 4 𝑓𝑜𝑓𝑥 = 44𝑥 − 36𝑥 − 4 − 364𝑥 − 36𝑥 − 4Area between y = x^2 and x = 1 and x = 3



How To Solve X 4 2x 3 3x 2 2x 1 0 Manually Quora




How To Find The Domain Of F X X 1 X 2 X 3 X 4 Quora



0 件のコメント:
コメントを投稿